I posed this puzzle a while back, and nobody solved it. That’s okay—now that I think about it, I’m not sure how to solve it either! 
It seems to involve group theory. But instead of working on it, solving it and telling you the answer, I’d rather dump all the clues in your lap, so we can figure it out together.
Suppose we have an ethyl cation. We’ll pretend it looks like this:
As I explained before, it actually doesn’t—not in real life. But never mind! Realism should never stand in the way of a good puzzle.
Continuing on in this unrealistic vein, we’ll pretend that the two black carbon atoms are distinguishable, and so are the five white hydrogen atoms. As you can see, 2 of the hydrogens are bonded to one carbon, and 3 to the other. We don’t care how the hydrogens are arranged, apart from which carbon each hydrogen is attached to. Given this, there are
ways to arrange the hydrogens. Let’s call these arrangements states.
Now draw a dot for each of these 20 states. Draw an edge connecting two dots whenever you can get from one state to another by having a hydrogen hop from the carbon with 2 hydrogens to the carbon with 3. You’ll get this picture, called the Desargues graph:
The red dots are states where the first carbon has 2 hydrogens attached to it; the blue ones are states where the second carbon has 2 hydrogens attached to it. So, each edge goes between a red and a blue dot. And there are 3 edges coming out of each dot, since there are 3 hydrogens that can make the jump!
Now, the puzzle is to show that you can also get the Desargues graph from a different kind of molecule. Any molecule shaped like this will do:
The 2 balls on top and bottom are called axial, while the 3 around the middle are called equatorial.
There are various molecules like this. For example, phosphorus pentachloride. Let’s use that.
Like the ethyl cation, phosphorus pentachloride also has 20 states… but only if count them a certain way! We have to treat all 5 chlorines as distinguishable, but think of two arrangements of them as the same if we can rotate one to get the other. Again, I’m not claiming this is physically realistic: it’s just for the sake of the puzzle.
Phosphorus pentachloride has 6 rotational symmetries, since you can turn it around its axis 3 ways, but also flip it over. So, it has
states.
That’s good: exactly the number of dots in the Desargues graph! But how about the edges? We get these from certain transitions between states. These transitions are called pseudorotations, and they look like this:
Phosphorus pentachloride really does this! First the 2 axial guys move towards each other to become equatorial. Beware: now the equatorial ones are no longer in the horizontal plane: they’re in the plane facing us. Then 2 of the 3 equatorial guys swing out to become axial.
To get from one state to another this way, we have to pick 2 of the 3 equatorial guys to swing out and become axial. There are 3 choices here. So, we again get a graph with 20 vertices and 3 edges coming out of each vertex.
Puzzle. Is this graph the Desargues graph? If so, show it is.
I read in some chemistry papers that it is. But is it really? And if so, why? David Corfield suggested a promising strategy. He pointed out that we just need to get a 1-1 correspondence between
• states of the ethyl cation and states of phosphorus pentachloride,
together with a compatible 1-1 correspondence between
• transitions of the ethyl cation and transitions of phosphorus pentachloride.
And he suggested that to do this, we should think of the split of hydrogens into a bunch of 2 and a bunch of 3 as analogous to the split of chlorines into a bunch of 2 (the ‘axial’ ones) and a bunch of 3 (the ‘equatorial’ ones).
It’s a promising idea. There’s a problem, though! In the ethyl cation, a single hydrogen hops from the bunch of 3 to the bunch of 2. But in a pseudorotation, two chlorines go from the bunch of 2 to the bunch of 3… and meanwhile, two go back from the bunch of 3 to bunch of 2.
And if you think about it, there’s another problem too. In the ethyl cation, there are 2 distinguishable carbons. One of them has 3 hydrogens attached, and one doesn’t. But in phosphorus pentachloride it’s not like that. The 3 equatorial chlorines are just that: equatorial. They don’t have 2 choices about how to be that way. Or do they?
Well, there’s more to say, but this should already make it clear that getting ‘natural’ one-to-one correspondences is a bit tricky… if it’s even possible at all!
If you know some group theory, we could try solving the problem using the ideas behind Felix Klein’s ‘Erlangen program’. The group of permutations of 5 things, say 
We could start by trying to see if 
I could say more, but I’ll stop here. In case you’re wondering, all this is just a trick to get more mathematicians interested in chemistry. A few may then go on to do useful things.
Azimuth 
Does it not become very natural if you label the hydrogens on the ethyl cation in a different way?
Instead of labelling them as which carbon they’re on ignore the carbons altogether and label them according to whether they’re in a set of 3 or a set of 2 (maybe set is the wrong word, I just thought it would be less confusing than “group” – interpret in the non-maths-y sense) – this is exactly the same labelling as for phosphorus pentachloride.
The pseudorotations and the hydrogen-swapping are then both just the action of turning the 3-set into a 2-set by moving one hydrogen. This is a little more difficult to see with the phosphorus pentachloride but the two equatorial hydrogens that you choose to physically “move” are the ones “staying in their set” and then one that stays physically stationary is the one that “swaps into” the other set.
No group theory needed, just thinking about them in a very slightly different way and it’s obvious.
Nice!!! That was fast!
I think there’s a wee bit more work to be done, though…
Labelling each hydrogen according to whether it’s in the set of 3 or the set of 2 does not completely specify a state of the ethyl cation. Since we’re treating the carbons are distinguishable, one extra bit of information is needed: which carbon has 3 hydrogens attached to it and which has 2?
Here ‘bit’ is used in the technical sense: binary digit. This extra bit is what brings the number of states up from
to
However, this bit of information always changes when we make a transition. Indeed, this bit says the color of the dots here:
and each edge goes between a red dot and a blue one.
Over on the phosphorus pentachloride side, there’s also an extra bit of information, in addition to the labelling saying which chlorines are axial and which are equatorial.
So, we’ve reduced the puzzle to this mini-puzzle: what is this extra bit, and does it always change when we do a pseudorotation? If it does, we’re done.
By the way, there’s another way of counting states in both molecules, where we ignore this extra bit. If we used that way, we’d have 10 states instead of 20… and we’d be done with this puzzle.
But there’s another fun question to ask about this other way of counting states: what graph would we get then?
Does the extra bit come from the reflectional symmetry of the phosphorus pentachloride? So, if a and b are axial, with a above, then the equatorial (b c d) clockwise is not the same as (d c b) clockwise.
Yes, this extra bit does not change when we rotate the phosphorus pentachloride molecule—see my reply to Peter Morgan below—but it does change when we reflect it. The question is whether it changes when we pseudorotate it!
Just thinking out loud.
Let me write [a,b,c,d,e] for the first image in this post labeled in reading order. So a and e are the axial atoms, bcd are equatorial in counter clockwise order. We have by rotational symmetry that: [a,b,c,d,e] = [a,c,d,b,e] = [a,d,b,c,e] = [e,d,c,b,a] = [e,c,b,d,a] = [e,b,d,c,a]. I will always use the lexicographically smallest representation.
Then the pseudorotation in the image in this post is [a,b,c,d,e] |-> [b,a,e,c,d].
According to a simple program I wrote, there are 10 different states reachable from [1,2,3,4,5] in an even number of moves:
[[1,2,3,4,5],[1,2,4,5,3],[1,2,5,3,4],[1,3,5,4,2],[2,1,3,5,4],[2,1,4,3,5],[2,1,5,4,3],[3,1,2,4,5],[3,1,5,2,4],[4,1,3,2,5]]
and 10 in an odd number of moves:
[[1,2,3,5,4],[1,2,4,3,5],[1,2,5,4,3],[1,3,4,5,2],[2,1,3,4,5],[2,1,4,5,3],[2,1,5,3,4],[3,1,2,5,4],[3,1,4,2,5],[4,1,2,3,5]]
The bit could be the parity of the permutation (a,b,c,d,e). That is because pseudorotation has odd parity, while all symmetry permutations have even parity.
———————————-
{-# LANGUAGE NoMonomorphismRestriction #-}
import Data.List
import qualified Data.Set as Set
perm xs = sort $ perm1 xs ++ perm1 (reverse xs)
perm1 [a,b,c,d,e] = [[a,b,c,d,e],[a,c,d,b,e],[a,d,b,c,e]]
cannon = head . perm
rot [a,b,c,d,e] = [b,a,e,c,d]
close :: Ord a => (a -> [a]) -> a -> Set.Set a
close f = go Set.empty
where
go s a
| a `Set.member` s = s
| otherwise = foldl go (Set.insert a s) (f a)
nextStates = map (cannon . rot) . perm
s0 = [1..5]
states = close nextStates s0
sEven = close (concatMap nextStates . nextStates) s0
sOdd = close (concatMap nextStates . nextStates) (nextStates s0!!0)
parity xs = (`mod`2) $ sum[ if x>y then 1 else 0 | x:ys <- tails xs, y<-ys ]
Excellent! The puzzle is solved!
More later… now it’s my bedtime. I’d like to try to find a way to solve this puzzle using plain English.
Good way of looking at it, Twan. Now, however, the pseudorotation looks to me to be a bit of a red herring as far as counting states is concerned. States are the same if they can be reached by rotations; rotations generate cycles containing 6 elements, [a,b,c,d,e] = [a,c,d,b,e] = [a,d,b,c,e] = [e,d,c,b,a] = [e,c,b,d,a] = [e,b,d,c,a], therefore there are 20 equivalence classes=states.
The pseudorotation plus *either* the rotation [a,b,c,d,e]->[a,c,d,b,e] *or* the rotation [a,b,c,d,e]->[e,b,d,c,a] generate cycles with 120 elements, with no grading. The pseudorotation plus the reflection [a,b,c,d,e]->[e,b,c,d,a] generates a cycle with 120 elements, with an odd-even grading. All of these facts have no bearing on the counting of states, but they show that the pseudorotation is sufficient to generate all transformations between the 20 equivalence classes under rotations.
There are also, a propos of nothing, 20 equivalence classes under pseudorotations, which generate cycles containing 6 elements, or 40 equivalence classes under even numbers of pseudorotations, which generate cycles containing 3 elements, [a,b,c,d,e] = [a,b,e,c,d] = [a,b,d,e,c].
I hope this rehearsal as I see it now looks OK. Thanks for the puzzle, John. Not sure yet how to present equivalence classes in plain English.
For the ethyl cation, a more-or-less comparable formalism has a list of two lists, one containing 2 elements, the other 3, [[1,2],[3,4,5]], which we take *not* to be equivalent to [[3,4,5],[1,2]]. On the other hand, the permutations of 3,4,5 and of 1,2 form an equivalence class of 12 elements. Hence, we have
states. Not sure how to introduce an equivalence to the phosphorus pentachloride case.
We get 20 in the ethyl cation case by “pretending” that the carbon atoms are distinguishable, so that we get the *2 in Binomial(5,2)*2 as the number of states, and the set of states is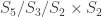
. Equally, we can “pretend” that the two axial positions in potassium pentachloride are distinguishable, so that the number of states is multinomial(5,1,1), and the set of states is 
. Alternatively, we can “pretend” that even and odd permutations of the equatorial positions in potassium pentachloride are distinguishable, so that the number of states is binomial(5,2)*2 and the set of states is 
.
In all three cases, other things being equal, all 20 states are equivalent to each other. You seem to be postulating a model dynamics of discrete moves between states at each discrete time step (without any of the probabilistic weighting that we’ve seen in earlier posts). Within a class of models of this type, we could reasonably define the ethyl cation model and the potassium pentachloride model as equivalent, as isolated discrete dynamical models, just if the single step transition graphs for the two discrete dynamics are, on some definition of graph equivalence, equivalent graphs (your geometrical description of the potassium pentachloride dynamics, however, introduces intermediate states that have a different symmetry structure, aiming at an equivalence of the ethyl cation dynamics with a subgraph of the potassium pentachloride dynamics, which has what I suppose could be called a
grading).
I suppose that for any sort of detailed modeling we will have to find ways to modify these structures to add the effect of another molecule, of an electromagnetic field, or of some other perturbation to the single-molecule model, and that the 20 states will then not be equivalent, or that the number of states might change as the model symmetries change. In any case, I hope that at some level of detail an empirically accurate model will distinguish between an ethyl cation and a potassium pentachloride molecule.
I’m not sure you’ll think this solves the puzzle?
Peter wrote:
Nice!!! But…
You just mentioned two very tempting ways to introduce an extra bit of information into the phosphorus pentachloride problem. But we don’t get to pick how to introduce that extra bit of information: the puzzle as stated tells us how we have to do it!
The puzzle says that two molecules of phosphorus pentachloride with chlorine atoms labelled 1,2,3,4,5 count as being in the same state if we can rotate one to the other, carrying the labelling of one to the labelling of the other.
So, the two axial positions are not distinguishable: we can always rotate the molecule so that the ‘top’ chlorine becomes the ‘bottom’ one, and vice versa.
Similarly, the two cyclic orderings of the equatorial chlorines are not distinguishable: we can always rotate the molecule so that the ‘clockwise’ cyclic ordering becomes the ‘counterclockwise’ one, and vice versa.
However, you’ll note that flipping the molecule over to make the top chlorine the bottom one also changes the clockwise cyclic ordering into the counterclockwise one.
So while neither of your ways of introducing an extra bit of information is the way prescribed by the problem, the sum of these two bits is. (Here I’m adding bits mod 2.)
So the mini-puzzle is whether this bit inevitably changes when we do a pseudorotation.
I was just about to work this out when I came back to my computer and saw your comment. It’s nice to see we’re thinking about the same stuff. This problem has been bugging me for weeks, but now it’s almost solved.
So, there are 40 states in the state space for the potassium pentachloride case, which contains a 20 state subspace that is closed under pairs of reflections. Pseudorotations are not rotations, but they are one (of many) paths that implement reflections. I note that the pseudorotations are paths that preserve the lengths of the potassium-chlorine bonds, but not, except at the endpoints, the angles between them.
My state space for phosphorus pentachloride has 20 states, not 40. I defined it here and also in Part 14. I’m sure you’re doing something interesting, but it’s dinner-time so I’ll read it later!
By the way it’s phosphorus, not potassium. Potassium can’t hang on to 5 chlorines!
Phosphorous-Potassium, Duh!
There’s a sense in which your state-space is infinite-dimensional, if you include pseudo-rotations as continuous paths between the 20.
I hope learning how to divide and multiply by 2 in different ways is doing me some good. Forget the 40.
Peter wrote:
Well, my plan for ‘Part 15’ of the Network Theory series is to look at:
• a Markov chain model, where time is discrete, and the molecule has probabilities to hop from one state to another at each time step,
and also
• a Markov process model, where time is continuous, and the molecule has ‘probabilistic rates’ of hopping from one state to another.
The second one is a bit more realistic, but the two models are mathematically related in a nice way. I also plan to look at quantum analogues of both models, where instead of probabilities we have amplitudes.
None of this is especially tied to the particular molecules I’m talking about now. We could start with any graph and study models of this sort. However, one nice feature of these particular molecules is that they’re so symmetrical that the transition probabilities, or rates, must be the same for every edge of the graph! This simplifies these models a bit… and if I went far enough, which I probably won’t, it’d let us use the representation theory of the group
to solve these models.
Yes, that’s the idea here: abstract away from the molecule and look at the graph.
In fact the real-world ethyl cation is nothing like what I’ve drawn here. As I explained in Part 14, it really looks like this:
However, transitions in the purely imaginary ethyl cation I discussed in this puzzle are easier to visualize than pseudorotations of phosphorus pentachloride! So, I’ll talk about ethyl cations that look like this:
even though they don’t exist. Translate the results to the phosphorus pentachloride picture, if you like…
Just a quick note…
Who is to say Markov processes are more realistic than Markov chains? Markov processes could represent continuum approximations to something that is fundamentally finitary.
maybe you should substitute a chlorine atom for the positive charge in the ethyl cation – think about ethyl chloride instead…
the trouble is that the three hydrogen atoms on the one carbon of the ethyl cation are *not* distinguishable (C3 symmetry), and the two hydrogens on the other carbon are not distinguishable either (C2 symmetry); similarly, the two axial chlorines on the PCl5 molecule have C2 symmetry, and the three equatorial chlorines have C3 symmetry, but the connectivity is different – in ethyl cation you’ve got a two-carbon chain, while in PCl5 you’ve got single P atom with 5 chlorine atoms on it. Substituents (chlorines, hydrogens, etc) tend to situate themselves so as to minimize Coulombic repulsions. Your picture with the “cyclic” C-C-H structure is an average – it makes the two carbons indistinguishable – they have “half-ownership” of one of the hydrogens – not the highly strained full ownership that the picture depicts.
Thanks for all the info, Hudson! Yes, Bacharach claims the ethyl cation actually looks like this:
and I’m treating all sorts of things as distinguishable that actually aren’t. So, this was a math puzzle with chemistry serving as a rather flimsy excuse, not an actual chemistry puzzle. Still it may get some mathematicians interested in graph theory problems coming from chemistry!
• Danail Bonchev and D.H. Rouvray, eds., Chemical Graph Theory: Introduction and Fundamentals, Taylor and Francis, 1991.
• Nenad Trinajstic, Chemical Graph Theory, CRC Press, 1992.
• R. Bruce King, Applications of Graph Theory Topology in Inorganic Cluster Coordination Chemistry, CRC Press, 1993.
The second one is apparently the magisterial tome of the subject. The prices on these books are absurd: for example, Amazon sells the first for $300, and the second for $222. Luckily some universities will have them…
The experimental evidence favours the non-classical structure.
Andrei, H.-S.; Solcà, N.; Dopfer, O., “IR Spectrum of the Ethyl Cation: Evidence for the Nonclassical Structure, Angew. Chem. Int. Ed. 2008, 47, 395-397
I looked at the Bacharach reference and see that his calculations represent the ethyl cation as an ethylene molecule, H2C=CH2, with a proton associated halfway between the two carbons, and bonded to neither. This gives the ethyl cation the same symmetry on average as ethylene, which I think is D2h, C2 with a mirror plane of symmetry between the two carbons (C2(z) C2(y) C2(x) i σ(xy) σ(xz) σ(yz)), whereas phosphorus pentachloride is D3h (2C3 3C2 σh 2S3 3σv)
If you decide on a way to uniquely identify states, you can match them up to your picture of the Desargues graph and each one will match either a blue or a red dot. So the extra bit is whether the state belongs to the blue dot set or the red dot set. This requires that you manually work out all of the possible transitions, but it does work. Unsatisfying perhaps.
I can also see a way to calculate a bit that will flip for each transition. (I teach high school physics and my group theory and abstract algebra is very rusty, so excuse me if I express things clumsily). Arrange the chlorine atoms in a cycle (1 2 3 4 5). The axial atoms are either adjacent in the cycle or not. Call that bit A.
Using the cycle we can assign any two atoms an order by requiring that the distance in the cycle between the earlier and later atoms is at most two. Rotate the atom so that the axis of symmetry is vertical and so that the top atom is the earlier of the two axial atoms. Then, looking down, the equatorial atoms are in clockwise order or not. Call that bit B.
The bit defined by A XOR B will flip in any transition.
I don’t have a good proof of this, but staring at some representative examples is convincing:
1
345
2
Here 1 and 2 are axial (and adjacent in the cycle) and 345 are equatorial in clockwise order looking down (to make the representation unique I’ll start with the smallest integer). There are exactly 3 possible transitions. If we replace 1 and 2 with two adjacent atoms (3 and 4 or 4 and 5), the equatorial order will reverse. If we replace them with the two non-adjacent atoms (3 and 5) then the equatorial order stays the same, but adjacency flips.
Another example, starting from non-adjacent axial atoms:
1
245
3
Again, flipping adjacency preserves equatorial order. If adjacency stays the same, order flips. I hope this makes sense to someone other than me.
In the second example, remember that 5 is earlier than 2 in the cycle.
There is a way to represent each of the 20 states of the ethyl cation as an ordered pair made with the numbers {1, 2, 3, 4, 5}. Also, there is a way to represent each of the 20 states of the phosphorus pentachloride as such an ordered pair. And the transitions correspond via these representations.
The key is given by this wu xing diagram:
An ordered pair corresponds to an arrow from the wu xing diagram, together with a sign: a plus sign telling that the pair has the same order as the arrow, or a minus sign if the order is the inverse one.
Let’s label the five hydrogen atoms of the the ethyl cation by the numbers {1, 2, 3, 4, 5}, and the two carbon ones by the plus and minus signs {+, -}. We associate to each of the 20 states the pair formed by the two numbers representing the two hydrogen atoms linked to the carbon with two hydrogen atoms. To find the order, we look up in the wu xing diagram the arrow labeled with the same numbers as the pair of hydrogen atoms. If the carbon atom is labeled with the plus sign, we order them by the order given by the arrow. If the carbon atom is labeled with the minus sign, we take the inverse order. For example, if the hydrogen atoms labeled with {1, 2, 3} are connected to the carbon atom labeled with the plus sign, and the hydrogen atoms labeled with {4, 5} are connected to the carbon atom labeled with the minus sign, the ordered pair used to represent the state of the molecule is (5, 4).
Let’s now represent the 20 states of the phosphorus pentachloride. We need first to define the sign of a triple in the wu xing diagram. It is made by the product between two signs. The first sign is given by the orientation of the triangle made by the pair: it is plus iff the orientation is counterclockwise. The second sign is given by minus one to the number of arrows from the triangle which are on the contour of the wu xing diagram. There are two kinds of triangles: the obtuse ones, with two arrows on the contour, and the acute ones, with one arrow on the contour. We multiply the signs and obtain the sign of the triple.
Back to the 20 states of the phosphorus pentachloride. We label with the same numbers {1, 2, 3, 4, 5} the five atoms. We represent the state by the pair given by the two axial atoms. The order is obtained by the following rule. We consider in the 3D representation of the molecule, a vector connecting the two axial atoms, oriented as in the wu xing diagram. With the wu xing diagram, we calculate the sign of the triple made by the three numbers labeling the equatorial atoms. We then multiply with the orientation of the triangle made by the three equatorial atoms in the 3D picture, seen from the direction in which the vector is pointing. If the resulting sign is plus, the pair representing the state is given by the orientation of the arrow connecting the labels of the two axial atoms. If it is minus, we reverse the order indicated by the arrow.
For example, let’s say that the bottom axial atom is labeled with 1, the top one with 2, and the three equatorial atoms are labeled in counterclockwise order with 3, 4, 5. The wu xing diagram shows that the arrow goes from 1 to 2. The orientation of the triangle made with 3, 4, 5 is clockwise in the wu xing diagram, and it is obtuse, so its sign is minus. Unlike the 3D diagram in which the sign is plus, the triangle being CCW. Therefore, the state should be represented by the pair (2, 1).
The next step is to see that the transitions coincide. The rule of the transition, common to the two types of molecules, is the following: an ordered pair can go in another ordered pair, if they don’t have common elements. In addition, we invert the sign. In other words, if a pair is oriented the same as the arrow represented it, then the transition will give a pair which is oriented in the inverse direction than that given by the arrow representing it, and conversely.
Cool! Why do you call that a “wu xing diagram”? Was some diagram like that used in China for some reason? My wife studies Chinese philosophy, religion, and history, so I’m curious. It looks almost like the Petersen graph:
but not quite, since the Petersen graph has 10 vertices instead of just 5.
Oh, I guessed it and confirmed it: wu xing refers to ‘five phase theory’, roughly the Chinese version of the Western ‘four elements’, although the phases were more mutable than elements.
Thanks! And you guessed well.
There is a simpler way to make the correspondence between the 20 states of the ethyl cation and those of the phosphorus pentachloride. The idea is that, instead of labeling the carbon atoms of the ethyl cation, we can use the two possible orientations of the three hydrogen atoms connected to the same carbon atom.
We start with a phosphorus pentachloride state. We replace the central atom with a carbon, and the five terminal atoms with hydrogenes. We draw the vector connecting the axial atoms, oriented as in the wu xing diagram. Then, we replace the axial atom towards which the vector points, with a carbon atom to which we link the two axial atoms. This operation transforms a phosphorus pentachloride state in a ethyl cation state, with the triple of hydrogen atoms oriented the same as in the phosphorus pentachloride state. We now can check that the transition diagrams are isomorphic.
P.S. I have thought at 1) one proof using a regular 5-cell in the four dimensional space, and hyperplanes separating its vertices, and 2) another one using the 20 vertices of a labeled dodecahedron which I constructed to represent the multiplication table of the even permutations of 5 elements (http://www.unitaryflow.com/2009/06/polyhedra-and-groups.html). I can write them down if you are interested, but I see that you already have enough proofs!
Just wondering: Earth absorbs water, water extinguishes fire, fire melts metal, metal cuts wood, but how does wood overcome earth?
Makes for a nice rock-paper-scissors game.
…ok, I looked it up: wood parts earth, as in tree roots cracking stone.
“Makes for a nice rock-paper-scissors game.”
Well, maybe because the rock-paper-scissors game is Chinese too (http://en.wikipedia.org/wiki/Rock_paper_scissor#History). Indeed, for 4 or 5 players the rock-paper-scissors game does not offer enough options to avoid repetitions, but wu-xing offers :)
It was sort of silly for me to post this puzzle both here and on the n-Category Café, but I was desperate for help. Here’s a really nice answer from Tracy Hall over at the n-Café. He answers the main puzzle and also my subsidiary puzzle, namely: what graph do we get if we discard the extra bit of information that says which carbon here has 3 hydrogens attached to it and which has 2?
The answer is the Petersen graph:
Tracy wrote:
Is there a nice place for the uninitiated to learn about cohomology of graphs?
Do you know about cohomology of anything else, like topological spaces or simplicial complexes? A graph is a special case of either of those.
I’m not trying to intimidate you by boosting the level of generality—honest. It’s just that if you happen to know some other kinds of cohomology, learning about the cohomology of graphs may be a lot easier than you think! But cohomology of graphs is, in fact, about the easiest kind of all.
If you only want to learn about the cohomology of graphs, you could learn it from the end of week293, where I apply it to electrical circuits, or from this book:
• P. Bamberg and S. Sternberg, A Course of Mathematics for Students of Physics vol. 2, Chap. 12: The theory of electrical circuits, Cambridge University, Cambridge, 1982.
which takes more time but goes into more detail.
Sometime fairly soon my Network Theory notes on this blog will get into electrical circuits, and then I’ll have to explain the cohomology of graphs.
Here’s a sketch of a solution that’s not too technical.
Puzzle. Show that the graph with states of a trigonal bipyramidal molecule as vertices and pseudorotations as edges is indeed the Desargues graph.
Answer. To be specific, let’s use iron pentacarbonyl as our example of a trigonal bipyramidal molecule:
It suffices to construct a 1-1 correspondence between the states of this molecule and those of the ethyl cation, such that two states of this molecule are connected by a transition if and only if the same holds for the corresponding states of the ethyl cation.
Here’s the key idea: the ethyl cation has 5 hydrogens, with 2 attached to one carbon and 3 attached to the other. Similarly, iron carbonyl has 5 carbonyl groups, with 2 axial and 3 equatorial. We’ll use this resemblance to set up our correspondence.
There are various ways to describe states of the ethyl cation, but here’s the best one for us. Number the hydrogens 1,2,3,4,5. Then a state of the ethyl cation consists of a partition of the set {1,2,3,4,5} into a 2-element set and a 3-element set, together with one extra bit of information, saying which carbon has 2 hydrogens attached to it. This extra bit is the color here:
What do transitions look like in this description? When a transition occurs, two hydrogens that belonged to the 3-element set now become part of the 2-element set. Meanwhile, both hydrogens that belonged to the 2-element set now become part of the 3-element set. (Ironically, the one hydrogen that hops is the one that stays in the 3-element set.) Moreover, the extra bit of information changes. That’s why every edge goes from a red dot to a blue one, or vice versa.
So, to solve the puzzle, we need to show that the same description also works for the states and transitions of iron pentacarbonyl!
In other words, we need to describe its states as ways of partitioning the set {1,2,3,4,5} into a 2-element set and a 3-element set, together with one extra bit of information. And we need its transitions to switch two elements of the 2-element set with two of the 3-element set, while changing that extra bit.
To do this, number the carbonyl groups {1,2,3,4,5}. The 2-element set consists of the axial ones, while the 3-element set consists of the equatorial ones. When a transition occurs, two of axial ones trade places with two of the equatorial ones, like this:
So, now we just need to figure out what that extra bit of information is, and why it always changes when a transition occurs!
Here’s how you calculate this extra bit. Hold the iron pentacarbonyl so that the axial guy with the lower number is pointing up, like this:
In this example the axial guys are numbered 2 and 4, so we hold the molecule so that the lower number, 2, is pointing up.
Then, looking down, see whether you can get the numbers of the three equatorial guys to increase as you read them going around clockwise, or counterclockwise. That’s your bit of information. In this example, you can read the numbers 1, 3, 5 as you go clockwise.
It’s easy to see that this bit of information doesn’t change when we rotate the iron carbonyl molecule, so we have a well-defined way of getting a bit from a state. On the other hand, this bit always changes when a transition occurs. For example:
At the end if you hold this molecule so the guy labelled 1 is pointing up, you can read the numbers 2, 4, 5 as you go around counterclockwise. So, the bit has changed.
This completes the proof except for checking that the bit always changes when a transition occurs. We leave this as a further small puzzle for the reader.
That bit doesn’t always change. Try the one with 1 and 2 as axial and 3, 4, 5 clockwise looking down. Make the transition to 3 and 5 as axial and you’ll get 1, 2, 4 clockwise looking down, so no bit change. It’s more complicated than this. I started out thinking like this, but ended up with what I posted in my last comment.
Ugh, you’re right! When try this example I start with
1
345
2
(in your notation)
and wind up with
3
234
5
Zounds!
It seems to me that there’s a necessary symmetry missing when you use the numerical order. Without it the relationship between up/down order and clockwise/counterclockwise order is inconsistent. Using a cycle and defining order in terms of shortest distance in the cycle (so 5 is after 3 but before 1 and 2) fixes this. But then there is also a difference if the axial atoms are consecutive in the cycle or 2 steps apart (those are the only two possibilities). If you think of that and the cw/ccw as bits, then one of those two bits flips in each transition. There’s probably a nicer way to formulate that but I think it’s correct.
Okay, I think the following solution is correct. This time I used Twan van Laarhoven’s idea for computing that extra bit of information. It not only works better than my earlier wrong approach; it’s also easy to give a full proof that it works.
Puzzle. Show that the graph with states of a trigonal bipyramidal molecule as vertices and pseudorotations as edges is indeed the Desargues graph.
Answer. To be specific, let’s use iron pentacarbonyl as our example of a trigonal bipyramidal molecule:
It suffices to construct a 1-1 correspondence between the states of this molecule and those of the ethyl cation, such that two states of this molecule are connected by a transition if and only if the same holds for the corresponding states of the ethyl cation.
Here’s the key idea: the ethyl cation has 5 hydrogens, with 2 attached to one carbon and 3 attached to the other. Similarly, the trigonal bipyramidal molecule has 5 carbonyl grops, with 2 axial and 3 equatorial. We’ll use this resemblance to set up our correspondence.
There are various ways to describe states of the ethyl cation, but this is the best for us. Number the hydrogens 1,2,3,4,5. Then a state of the ethyl cation consists of a partition of the set {1,2,3,4,5} into a 2-element set and a 3-element set, together with one extra bit of information, saying which carbon has 2 hydrogens attached to it. This extra bit is the color here:
What do transitions look like in this description? When a transition occurs, two hydrogens that belonged to the 3-element set now become part of the 2-element set. Meanwhile, both hydrogens that belonged to the 2-element set now become part of the 3-element set. (Ironically, the one hydrogen that hops is the one that stays in the 3-element set.) Moreover, the extra bit of information changes. That’s why every edge goes from a red dot to a blue one, or vice versa.
So, to solve the puzzle, we need to show that the same description also works for the states and transitions of iron pentacarbonyl!
In other words, we need to describe its states as ways of partitioning the set {1,2,3,4,5} into a 2-element set and a 3-element set, together with one extra bit of information. And we need its transitions to switch two elements of the 2-element set with two of the 3-element set, while changing that extra bit.
To do this, number the carbonyl groups 1,2,3,4,5. The 2-element set consists of the axial ones, while the 3-element set consists of the equatorial ones. When a transition occurs, two of axial ones trade places with two of the equatorial ones, like this:
So, now we just need to figure out what that extra bit of information is, and why it always changes when a transition occurs.
Here’s how we calculate that extra bit. Hold the iron pentacarbonyl molecule vertically with one of the equatorial carbonyl groups pointing to your left. Remember, the carbonyl groups are numbered. So, write a list of these numbers, say (a,b,c,d,e), where a is the top axial one, b,c,d are the equatorial ones listed in counterclockwise order starting from the one pointing left, and e is the bottom axial one. This list is some permutation of the list (1,2,3,4,5). Take the sign of this permutation to be our bit!
Let’s do an example:
Here we get the list (2,5,3,1,4) since 2 is on top, 4 is on bottom, and 5,3,1 are the equatorial guys listed counterclockwise starting from the one at left. The list (2,5,3,1,4) is an odd permutation of (1,2,3,4,5), so our bit of information is odd.
Of course we must check that this bit is well-defined: namely, that it doesn’t change if we rotate the molecule. Rotating it a third of a turn gives an even permutation of the equatorial guys and leaves the axial ones alone, so this is an even permutation. Flipping it over gives an odd permutation of the equatorial guys, but it also gives an odd permutation of the axial ones, so it too is an even permutation. So, rotating the molecule doesn’t change the sign of the permutation we compute from it. The sign is thus a well-defined function of the state of the molecule.
Next we must to check that this sign changes whenever our molecule undergoes a transition. For this we need to check that any transition changes our list of numbers by an odd permutation. Since all transitions are conjugate in the permutation group, it suffices to consider one example:
Here we started with a state giving the list (2,5,3,1,4). The transition took us to a state that gives the list (3,5,4,2,1) if we hold the molecule so that 3 is pointing up and 5 to the left. The reader can check that going from one list to another requires an odd permutation. So we’re done.
I made a system of labels for each molecule:
Label the hydrogens of the ethyl cation with letters a through e. The state is labeled with the hydrogen pair and an orientation – if it was ab – cde, this is ab+. If it was cde – ab, label as ab-.
Fill out the graph this way, and label transitions (edges) with the hydrogen that changes place.
For the pentachloride we’ll have a similar system. Label each of the outer atoms with letters a through e. Each state gets a label that is the axis pair, in alphabetical order. Use alphabetical order of the axial atoms to determine an orientation – up or down. If the ordering of the axis matches the orientation, it’s +. If it does not, it’s -.
Psuedorotations will be labeled by the axial atom that does not participate.
Using the diagram for the original system, plot the edges and their labels on a second graph. Put the ab+ state in the same position as the original graph. Now, just go around using the moves already written as psuedorotations. These will be valid psuedorotations and will not repeat. If you fill in the missing edges, they’ll match the original graph.
This does not distinguish the states of the pentachloride into two sets the way the original system did – the original graph alternates the +/- around the graph (blue/red states), while this one does not.
It’s possible some similar system would fix this, but I’m done with it for now.